Bài 12 trang 213 SBT đại số 10

Giải các bất phương trình sau

LG a

\(\left| {x - 2} \right| < 2{x^2} - 9x + 9\)

Lời giải chi tiết:

TH1: \(x - 2 \ge 0\) \( \Leftrightarrow x \ge 2\) bất phương trình là:

\(\begin{array}{l}x - 2 < 2{x^2} - 9x + 9\\ \Leftrightarrow 2{x^2} - 10x + 11 > 0\\ \Leftrightarrow \left[ \begin{array}{l}x > \dfrac{{5 + \sqrt 3 }}{2}\\x < \dfrac{{5 - \sqrt 3 }}{2}\end{array} \right.\end{array}\)

Kết hợp \(x \ge 2\) ta được \(x > \dfrac{{5 + \sqrt 3 }}{2}\)

TH2: \(x - 2 < 0\) \( \Leftrightarrow x < 2\) bất phương trình là:

\(\begin{array}{l} - x + 2 < 2{x^2} - 9x + 9\\ \Leftrightarrow 2{x^2} - 8x + 7 > 0\\ \Leftrightarrow \left[ \begin{array}{l}x > \dfrac{{4 + \sqrt 2 }}{2}\\x < \dfrac{{4 - \sqrt 2 }}{2}\end{array} \right.\end{array}\)

Kết hợp \(x < 2\) ta được \(x < \dfrac{{4 - \sqrt 2 }}{2}\)

Vậy bpt có tập nghiệm \(S = \left( { - \infty ;\dfrac{{4 - \sqrt 2 }}{2}} \right) \cup \left( {\dfrac{{5 + \sqrt 3 }}{2}; + \infty } \right)\).

LG b

\({x^2} + 4 \ge \left| {3x + 2} \right| - 7x\)

Lời giải chi tiết:

TH1: \(3x + 2 \ge 0 \Leftrightarrow x \ge  - \dfrac{2}{3}\)

BPT trở thành

\(\begin{array}{l}{x^2} + 4 \ge 3x + 2 - 7x\\ \Leftrightarrow {x^2} + 4 \ge  - 4x + 2\\ \Leftrightarrow {x^2} + 4x + 2 \ge 0\\ \Leftrightarrow \left[ \begin{array}{l}x \ge  - 2 + \sqrt 2 \\x \le  - 2 - \sqrt 2 \end{array} \right.\end{array}\)

Kết hợp \(x \ge  - \dfrac{2}{3}\) ta được \(x \ge  - 2 + \sqrt 2 \).

TH2: \(3x + 2 < 0 \Leftrightarrow x <  - \dfrac{2}{3}\)

BPT trở thành

\(\begin{array}{l}{x^2} + 4 \ge  - 3x - 2 - 7x\\ \Leftrightarrow {x^2} + 4 \ge  - 10x - 2\\ \Leftrightarrow {x^2} + 10x + 6 \ge 0\\ \Leftrightarrow \left[ \begin{array}{l}x \ge  - 5 + \sqrt {19} \\x \le  - 5 - \sqrt {19} \end{array} \right.\end{array}\)

Kết hợp \(x <  - \dfrac{2}{3}\) ta được \(x \le  - 5 - \sqrt {19} \).

Vậy bpt có tập nghiệm \(S = \left( { - \infty ; - 5 - \sqrt {19} } \right] \cup \left[ { - 2 + \sqrt 2 ; + \infty } \right)\).

LG c

\(\dfrac{{2x + 3}}{{{x^2} + x - 12}} \le \dfrac{1}{2}\)

Lời giải chi tiết:

\(\begin{array}{l}BPT \Leftrightarrow \dfrac{{2x + 3}}{{{x^2} + x - 12}} - \dfrac{1}{2} \le 0\\ \Leftrightarrow \dfrac{{2\left( {2x + 3} \right) - \left( {{x^2} + x - 12} \right)}}{{2\left( {{x^2} + x - 12} \right)}} \le 0\\ \Leftrightarrow \dfrac{{ - {x^2} + 3x + 18}}{{{x^2} + x - 12}} \le 0\end{array}\)

Ta có: \( - {x^2} + 3x + 18 = 0\) \( \Leftrightarrow \left[ \begin{array}{l}x = 6\\x =  - 3\end{array} \right.\)

\({x^2} + x - 12 = 0\) \( \Leftrightarrow \left[ \begin{array}{l}x =  - 4\\x = 3\end{array} \right.\)

Xét dấu vế trái:

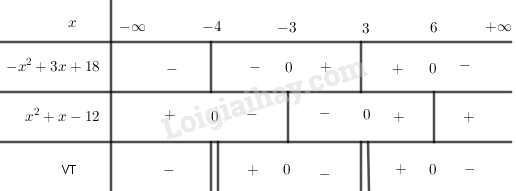
Từ bảng xét dấu ta thấy \(f\left( x \right) \le 0 \Leftrightarrow \left[ \begin{array}{l}x <  - 4\\ - 3 \le x < 3\\x \ge 6\end{array} \right.\)

Vậy tập nghiệm của bpt là \(\left( { - \infty ; - 4} \right) \cup \left[ { - 3;3} \right) \cup \left[ {6; + \infty } \right)\).

LG d

\(\dfrac{{{x^4} - 3{x^3} + 2{x^2}}}{{{x^2} - x - 30}} > 0\)

Lời giải chi tiết:

\(\begin{array}{l}\dfrac{{{x^4} - 3{x^3} + 2{x^2}}}{{{x^2} - x - 30}} > 0\\ \Leftrightarrow \dfrac{{{x^2}\left( {{x^2} - 3x + 2} \right)}}{{{x^2} - x - 30}} > 0\end{array}\)

Ta có: \({x^2} = 0 \Leftrightarrow x = 0\)

\({x^2} - 3x + 2 = 0 \Leftrightarrow \left[ \begin{array}{l}x = 1\\x = 2\end{array} \right.\)

\({x^2} - x - 30 = 0\) \( \Leftrightarrow \left[ \begin{array}{l}x = 6\\x =  - 5\end{array} \right.\)

Bảng xét dấu vế trái:

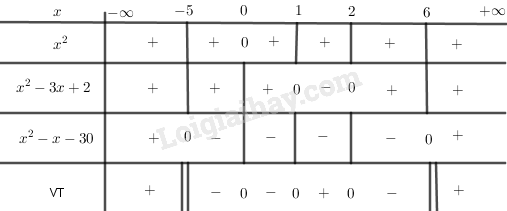
Từ bảng xét dấu suy ra \(f\left( x \right) > 0 \Leftrightarrow \left[ \begin{array}{l}x <  - 5\\1 < x < 2\\x > 6\end{array} \right.\)

Vậy bpt có tập nghiệm \(S = \left( { - \infty ; - 5} \right) \cup \left( {1;2} \right) \cup \left( {6; + \infty } \right)\).