Bài 4 trang 34 SGK Hình học 10 Nâng cao

Cho tam giác \(ABC\).

LG a

Tìm các điểm \(M\) và \(N\) sao cho

\(\overrightarrow {MA}  - \overrightarrow {MB}  + \overrightarrow {MC}  = \overrightarrow 0 \) và \(2\overrightarrow {NA}  + \overrightarrow {NB}  + \overrightarrow {NC}  = \overrightarrow 0 .\)

Lời giải chi tiết:

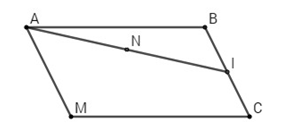
Ta có \(\overrightarrow {MA}  - \overrightarrow {MB}  + \overrightarrow {MC}  = \overrightarrow 0 \)

\(\Leftrightarrow \,\overrightarrow {BA}  + \overrightarrow {MC}  = \overrightarrow 0 \)

\(\begin{array}{l}
\Leftrightarrow \overrightarrow {BA} = - \overrightarrow {MC} \\
\Leftrightarrow \overrightarrow {BA} = \overrightarrow {CM}
\end{array}\)

Do đó \(ABCM\) là hình bình hành.

Gọi \(I\) là trung điểm của \(BC\), ta có \(\overrightarrow {NB}  + \overrightarrow {NC}  = 2\overrightarrow {NI} \) suy ra \(2\overrightarrow {NA}  + 2\overrightarrow {NI}  = \overrightarrow 0 \)

\( \Rightarrow \,\,\overrightarrow {NA}  + \overrightarrow {NI}  = \overrightarrow 0 \)

\(\Rightarrow \,N\,\) là trung điểm của \(AI\).

LG b

Với các điểm \(M, N\) ở câu a) , tìm các số \(p\) và \(q\) sao cho

\(\overrightarrow {MN}  = p\overrightarrow {AB}  + q\overrightarrow {AC} .\)

Phương pháp giải:

- Biểu diễn \(\overrightarrow {AM} ,\,\overrightarrow {AN} \) qua \(\overrightarrow {AB} ,\,\overrightarrow {AC} \).

- Từ đó suy ra véc tơ MN.

Lời giải chi tiết:

Từ câu a), ta biểu diễn \(\overrightarrow {AM} ,\,\overrightarrow {AN} \) qua \(\overrightarrow {AB} ,\,\overrightarrow {AC} \).

Do ABCM là hình bình hành nên:

\(\overrightarrow {AM}  = \overrightarrow {BC}  = \overrightarrow {AC}  - \overrightarrow {AB} \)

Do N là trung điểm AI nên:

\(\begin{array}{l}
\overrightarrow {AN} = \frac{1}{2}\overrightarrow {AI} = \frac{1}{2}.\frac{1}{2}\left( {\overrightarrow {AB} + \overrightarrow {AC} } \right)\\
= \frac{1}{4}\left( {\overrightarrow {AB} + \overrightarrow {AC} } \right) = \frac{1}{4}\overrightarrow {AB} + \frac{1}{4}\overrightarrow {AC}
\end{array}\)

(vì I là trung điểm BC nên \(\overrightarrow {AI}  = \frac{1}{2}\left( {\overrightarrow {AB}  + \overrightarrow {AC} } \right)\))

Do đó,

\(\begin{array}{l}
\overrightarrow {MN} = \overrightarrow {AN} - \overrightarrow {AM} \\
= \frac{1}{4}\overrightarrow {AB} + \frac{1}{4}\overrightarrow {AC} - \overrightarrow {AC} + \overrightarrow {AB} \\
= \frac{5}{4}\overrightarrow {AB} - \frac{3}{4}\overrightarrow {AC} \\
\Rightarrow \overrightarrow {MN} = \frac{5}{4}\overrightarrow {AB} - \frac{3}{4}\overrightarrow {AC}
\end{array}\)

Vậy \(p = {5 \over 4}\,;\,q =  - {3 \over 4}.\)