Bài 1.57 trang 44 SBT hình học 10

Đề bài

Cho tam giác \(ABC\). Gọi \(M, N , P\) là những điểm được xác định như sau:\(\overrightarrow {MB}  = 3\overrightarrow {MC} ,\overrightarrow {NC}  = 3\overrightarrow {NA} ,\)\(\overrightarrow {PA}  = 3\overrightarrow {PB} \)

a) Chứng minh \(2\overrightarrow {OM}  = 3\overrightarrow {OC}  - \overrightarrow {OB} \) với mọi điểm \(O\).

b) Chứng minh hai tam giác \(ABC\) và \(MNP\) có cùng trọng tâm.

Lời giải chi tiết

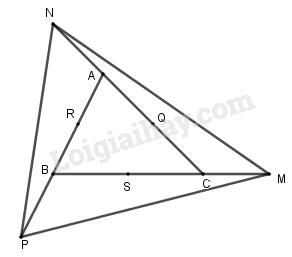
a) Ta có: \(3\overrightarrow {OC}  - \overrightarrow {OB} \)\( = 3(\overrightarrow {OM}  + \overrightarrow {MC} ) - (\overrightarrow {OM}  + \overrightarrow {MB} )\)

\( = 3(\overrightarrow {OM}  - \overrightarrow {OM} ) + (3\overrightarrow {MC}  - \overrightarrow {MB} )\)\( = 2\overrightarrow {OM} \)

b) Gọi \(S, Q\) và \(R\) lần lượt là trung điểm của \(BC, CA\) và \(AB\).

\(\overrightarrow {MB}  = 3\overrightarrow {MC}  \Rightarrow \overrightarrow {CM}  = \overrightarrow {SC} \); \(\overrightarrow {NC}  = 3\overrightarrow {NA}  \Rightarrow \overrightarrow {AN}  = \overrightarrow {CQ} \)

\(\overrightarrow {PA}  = 3\overrightarrow {PB}  \Rightarrow \overrightarrow {BP}  = \overrightarrow {RB}  = \overrightarrow {QS} \)

Gọi \(G\) là trọng tâm của tam giác \(ABC\) thì \(\overrightarrow {GA}  + \overrightarrow {BG}  + \overrightarrow {GC}  = \overrightarrow 0 \)

Ta có:

\(\overrightarrow {GM}  + \overrightarrow {GN}  + \overrightarrow {GP} \)\( = \overrightarrow {GC}  + \overrightarrow {CM}  + \overrightarrow {GA}  + \overrightarrow {AN}  + \overrightarrow {GB}  + \overrightarrow {BP} \)

\( = \left( {\overrightarrow {GA}  + \overrightarrow {GC}  + \overrightarrow {GC} } \right) + \left( {\overrightarrow {SC}  + \overrightarrow {CQ}  + \overrightarrow {QS} } \right)\)\( = \overrightarrow 0  + \overrightarrow 0  = \overrightarrow 0 \)

Vậy \(G\) là trọng tâm của tam giác \(MNP\).